*Department of Mechanical Engineering, School of Engineering & Computing Sciences, New York Institute of Technology,
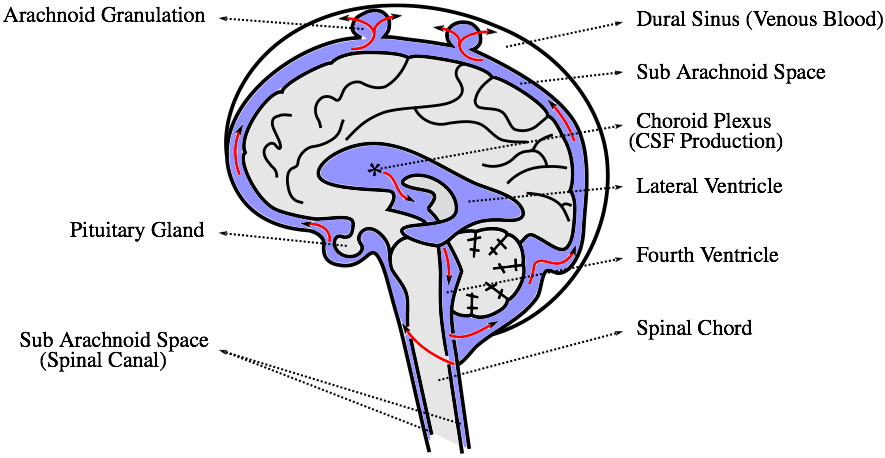
Old Westbury Campus - HSH 116A, Northern Boulevard, Old Westbury, New York 11568-8000, USA **Emory University, 201 Dowman Dr, Atlanta, GA 30322, USA

Abstract

If you have been involved in a car accident, whiplash injuries need to be taken very seriously. What protects your brain from severe injuries in such scenarios is the cerebrospinal fluid (CSF). The primary function of CSF is to cushion the brain within the skull and serve as a shock absorber for the central nervous system. In this study we observe this function using a comprehensive head model and advanced fluid-structure interaction (FSI) simulations with realistic whiplash boundary and initial conditions.

1 Introduction

Many reports focus on high speed motor vehicle collisions due to the large amount of property damage; therefore, injuries resulting from low speed collisions, especially brain injury from whiplash, are often overlooked. The neurological symptoms of whiplash, such as attention and memory issues, vertigo, and confusion, may not develop until weeks after the accident and are more difficult to treat than the physical aspect of pain. A detailed simulation of the brain injury due to whiplash may help identify the brain injury and therefore will aid in the treatment.

While the significance of including CSF in the numerical simulations has been shown [1], the current finite element studies reported in the literature often lack more detailed anatomical structures [2, 3, 4, 5]. More specifically, the CSF is commonly present only outside the brain, i.e. between the skull and one massive clump representing the brain. However, the brain is of more complicated structure, it consists of three main parts, namely the cerebrum, cerebellum, and brainstem (Fig. 1).

Figure 1: The schematic of the cerebrospinal fluid in which the brain is submerged. The 3D computational model used is designed based on this schematic.

Moreover, the cerebrum consists of two cerebral hemispheres, equal halves of the brain, and is positioned over and around most other brain structures. Each cerebral hemisphere is divided into four lobes by sulci and gyri. The sulci are the grooves and the gyri are the bumps that can be seen on the surface of the brain. The CSF fills a system of cavities at the centre of the brain, known as ventricles, and the subarachnoid space surrounding the brain and the spinal cord (Fig. 1).

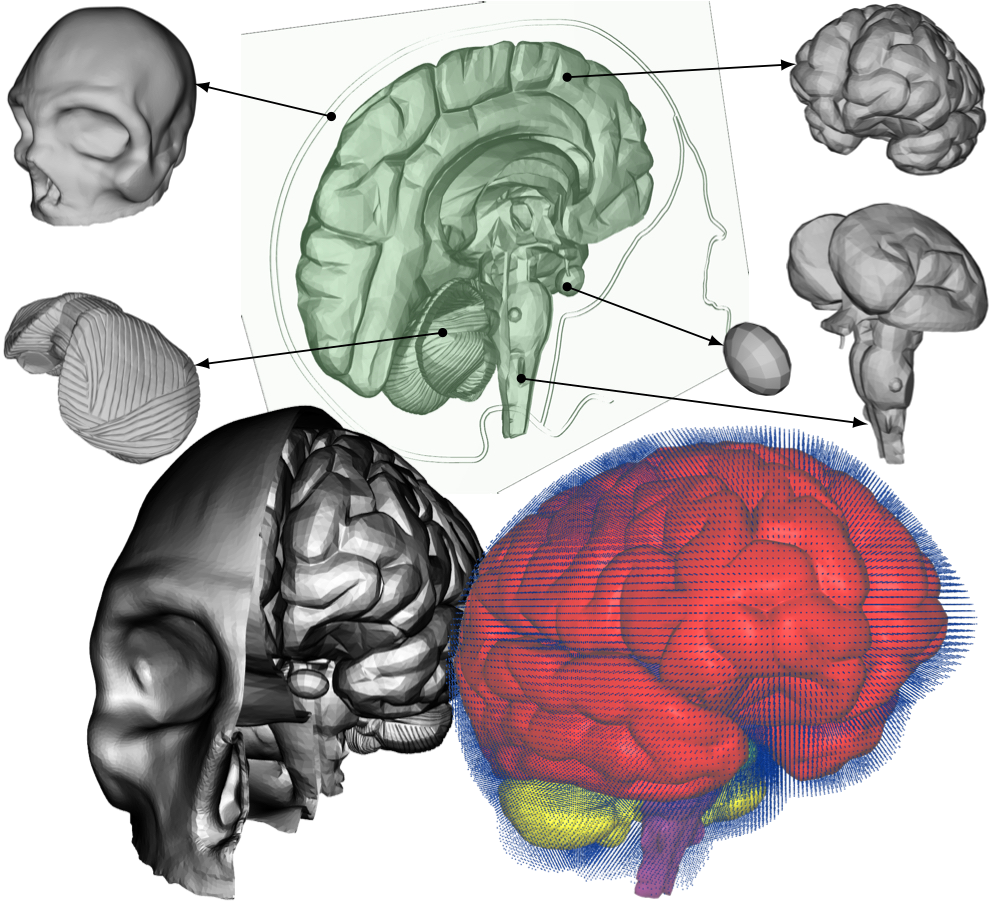
In this study, we present fluid-structure interaction analysis of a realistic head/brain model exposed to realistic conditions typical to car crash accidents. Over half of all reported traumatic brain injuries are the result of an automobile accident. There are other similar types of traumatic brain injuries in which the brain is bruised, e.g. coup [6] and contrecoup [7] injuries, which happen when a moving object impacts the stationary head or when the moving head strikes a stationary object, respectively. The exact mechanism for the brain injuries is a subject of much debate [8]. Complete understanding of the cushioning effect of the CSF is therefore still elusive and a detailed analysis of this function is axiomatic to the treatment and prevention of brain injuries.

2 Methodology

2.1 Crash conditions

To simulate the crash conditions later prescribed to our comprehensive head model, the HUMOS2 (HUman MOdel for Safety) [9] is used in the car crash sled test with a 3-point seat belt simulated using RADIOSS (Atlair Engineering, Michigan, USA). The HUMOS2 is a human numerical model based on real human data. It includes skeleton, muscles, organs, and ligaments. The acceleration/deceleration values of the HUMOS2's head from the sled test are then used to prescribe the velocity to a more comprehensive head model described below.

2.2 Head model

The velocity extracted from the above sled test simulation using a human numerical model is used to test the interaction of the CSF and brain/skull using a more comprehensive head model. The model of the head, namely skull, cerebrum, cerebellum, brainstem and pituitary gland, is shown in Fig. 2. The model is based on the digital imaging and communications in medicine (DICOM) images; thus, making it patient specific. The only major anatomical features missing in this model are the meninges, i.e. the three membranes that envelop the brain and spinal cord to protect the central nervous system. The arachnoid granulation, depicted in Fig. 1, is neglected, too. The fluid in the very short time period during which the accident scenario occurs can be considered static, therefore its flow does not need to be accounted for. Also, it is reasonable to assume that the flow of the CSF itself does not significantly contribute to the cushioning effect of the CSF. Both of these assumptions make the presence of the granulations negligible.

Figure 2: The depiction of the entire head model with skull, cerebrum, cerebellum, pituitary gland and brainstem, respectively. The subarachnoid space and other cavities are filled with fluid particles (blue dots surrounding the brain model, in the lower right corner). The entire model with half the skull is also shown (lower left).

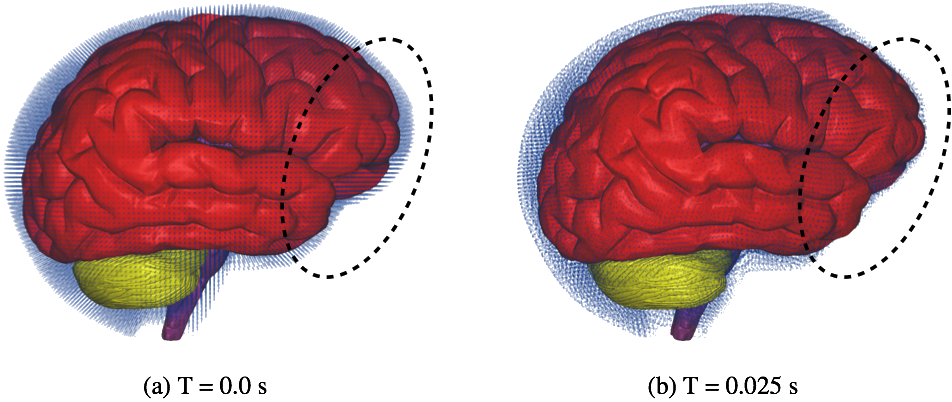
2.3 Computer Simulations

Fluid motion and boundary interaction were solved with the the IMPETUS Afea SPH Solver, while large deformation in the solid parts was simultaneously solved with the IMPETUS Afea Solver. Both the solvers use a commodity GPU for parallel processing. All solid elements were fully integrated removing the possibility of hourglass modes and element inversion that plagues the classic under-integrated elements. Both fluid and solid domains and their interaction were solved with an explicit integration scheme. All simulations were solved on a standard workstation. Parallel acceleration was achieved with a Tesla K40 GPU with 12 GB of Graphic DDR memory and 2880 CUDA Cores. To confirm that convergence was reached, h-refinement of the finite element mesh was performed and the solution was found to yield same results. The SPH equations are described in more details in our previous work [10]. We have opted to use the SPH method because using traditional fluid-structure interaction (FSI) techniques can still be computationally expensive and challenging when it comes to their parallelization [11]. In order to use the traditional FSI techniques, the complexity of the geometry used would have to be sacrificed.

3 RESULTS AND CONCLUSIONS

Relative displacement of the fluid particles compared to the brain can be observed. It can be seen that the fluid particles located in the front lobe have been pushed by the motion of the brain and thus allowing the brain in that region to come to contact with the skull, see inside the dashed ellipses in Fig. 3. The impact of the brain against the skull, and the many sharp edges, shelves, and protrusions on its inner surface, is known to be directly related to brain-associated problems after whiplash.

Figure 3: At T = 0.025 s, the skull reached the speed of 9 m·s−1 (red color), compared to the initial relative position of the fluid particles to the brain it can be seen that the front of the brain is not protected with the same thickness of CSF and, in fact, is in contact with the skull.

Using this methodology, the contact of the brain with the skull despite the presence of the CSF can be observed up to certain speed and the probability of brain injury can be subsequently assessed by measuring the stress values on the brain. The overall effect and function of the CSF can be analyzed. No symptom should be taken lightly in the event of a whiplash injury, especially that some of the symptoms may not develop until weeks after the accident. Therefore, our understanding of the mechanism of whiplash injury is axiomatic.

REFERENCES

[1] J. Vorwerk, M. Clerc, M. Burger, and C.H. Wolters,Comparison of boundary element and finite element approaches to the eeg forward problem, Biomedical Engineering / Biomedizinische Technik, vol. 57, no. SI-1 Track-O, pp. 795-798, 2012.
[2] Y. Luo, Z. Li, and H. Chen, Finite-element study of cerebrospinal fluid in mitigating closed head injuries, J. Engineering in Medicine, vol. 226, no. 7, pp. 499-509, 2012.
[3] M. Sotudeh Chafi, V. Dirisala, G. Karami, and M. Ziejewski, A finite element method parametric study of the dynamic response of the human brain with different cerebrospinal fluid constitutive properties, J. Engineering in Medicine, vol. 223, pp. 1003-1019, 2009.
[4] Z. Liang and Y. Luo, A qct-based nonsegmentation finite element head model for studying traumatic brain injury, Applied Bionics and Biomechanics, vol. 2015, pp. 1-8, 2015.
[5] L. Bei, R. Shijie, L. Haiyan, C. Shihai, and H. Lijuan, The effects of different mesh density of the cerebrospinal fluid on the dynamic responses of a 6 years old child finite element head model, in Eighth International Conference on Measuring Technology and Mechatronics Automation (ICMTMA), 2016, pp. 756-767.
[6] A.L. Morrison, T.M. King, M.A. Korell, J.E. Smialek, and J.C. Troncosso, Acceleration-deceleration injuries to the brain in blunt force trauma, American Journal of Forensic Medical Pathology, vol. 19, no. 2, pp. 109-112, 1998.
[7] M.P. Poirier, Concussions: Assessment, management, and recommendations for return to activity, Clinical Pediatric Emergency Medicine, vol. 4, no. 3, pp. 179-185, 2003.
[8] N.A. Shaw, The neurophysiology of concussion, Progress in Neurobiology, vol. 67, no. 4, pp. 281-344, 2002.
[9] M. Toma, F.E.A. Njilie, M. Ghajari, and U. Galvaneto, Assessing motorcycle crash-related head injuries using finite element simulations, Int. J. Simul. Model, vol. 9, no. 3, pp. 143-151, 2010.
[10] M. Toma, D.R. Einstein, C.H. Bloodworth, R.P. Cochran, A.P. Yoganathan, and K.S. Kunzelman, Fluid-structure interaction and structural analyses using a comprehensive mitral valve model with 3D chordal structure, Int. J. Numer. Meth. Biomed. Engng., 2016.
[11] M. Toma, M. Oshima, and S. Takagi, Decomposition and parallelization of strongly coupled fluid-structure interaction linear subsystems based on the Q1/P0 discretization, Computers & Structures, vol. 173, pp. 84-94, 2016.